Question
Determine the number of positive integers which are factor of the number: 34 x 52 x...
Determine the number of positive integers which are factor of the number: 34 x 52 x 117 x 132
Homework Answers
Answer #1
- This question uses one of the number theoretic functions
I.e.
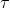 (n) called tao of n
which helps us to directly compute the number of positive divisors
of any number, however large it may be provided you can write it's
prime factorisation.
(n) called tao of n
which helps us to directly compute the number of positive divisors
of any number, however large it may be provided you can write it's
prime factorisation. 
- Thus, the number of divisors are 288.
Not the answer you're looking for?
Similar Questions
Let N denote the set of positive integers, and let x be a number which does...
Let N denote the set of positive integers, and let x be a number
which does not belong to N. Give an explicit bijection f : N ∪ x →
N.
Determine, with proof, the largest number that is the product of positive integers whose sum is...
Determine, with proof, the largest number that is the product of
positive integers whose sum is 1976.
Prove that there exist n consecutive positive integers each having a (nontrivial) square factor. How would...
Prove that there exist n consecutive positive integers each
having a (nontrivial) square factor. How would you then modify your
proof so that each of these integers instead has a cube factor (or
more generally, a kth power factor where k ≥ 2)?
This is a number theory question.
Please show all steps and make clear notes about what is happening
for a clear understanding.
Please write clearly or do in latex.
Thank you
For some positive integers t, y + 5 = (1/t) * (x^2) intersects x^2 + y^2...
For some positive integers t, y + 5 = (1/t) * (x^2) intersects
x^2 + y^2 = 25 at exactly 3 points P, Q, and R (distinct from one
another). Determine the positive integers t for which the area of
triangle PQR is an integer.
Let X be a subset of the integers from 1 to 1997 such that |X|≥34. Show...
Let X be a subset of the integers from 1 to 1997 such that
|X|≥34. Show that there exists distinct a,b,c∈X and distinct
x,y,z∈X such that a+b+c=x+y+z and {a,b,c}≠{x,y,z}.
Find the number of positive integers ≤ 1000 that are multiples of at least one of...
Find the number of positive integers ≤ 1000 that are multiples
of at least one of 3, 5, 11.
Find all positive integers x and y that satisfy x2 - y2= 2018? Are there any...
Find all positive integers x and y that satisfy x2 -
y2= 2018? Are there any positive integers x and y such
that x3 − y3 = 2018? Explain.
Find the number of positive integers not exceeding 10,000 that are not divisible by 3, 4,...
Find the number of positive integers not exceeding 10,000 that
are not divisible by 3, 4, 7, or 11.
Prove that for fixed positive integers k and n, the number of partitions of n is...
Prove that for fixed positive integers k and n, the number of
partitions of n is equal to the number of partitions of 2n + k into
n + k parts.
show by using bijection
Determine all values of n for which the following statement is true: There exists integers x...
Determine all values of n for which the following statement is
true: There exists integers x and y such that 63x + 147y = n.
Give a convincing argument to justify your answer.
ADVERTISEMENT
Need Online Homework Help?
Get Answers For Free
Most questions answered within 1 hours.
ADVERTISEMENT
Active Questions
asked 9 minutes ago
asked 13 minutes ago
asked 15 minutes ago
asked 42 minutes ago
asked 44 minutes ago
asked 1 hour ago
asked 1 hour ago
asked 1 hour ago
asked 1 hour ago
asked 1 hour ago
asked 1 hour ago
asked 1 hour ago
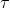 (n) called tao of n
which helps us to directly compute the number of positive divisors
of any number, however large it may be provided you can write it's
prime factorisation.
(n) called tao of n
which helps us to directly compute the number of positive divisors
of any number, however large it may be provided you can write it's
prime factorisation.